Let's see how to derive equation of tangent line when we are given equation of curve r = f ( θ ) {r}={f{{\left(\theta\right)}}} r = f ( θ )
We will proceed in the same fashion as with tangent lines to parametric curves , because polar coordinates in some sense similar to parametric curves.
We know that x = r cos ( θ ) {x}={r}{\cos{{\left(\theta\right)}}} x = r cos ( θ ) y = r sin ( θ ) {y}={r}{\sin{{\left(\theta\right)}}} y = r sin ( θ )
Since we are given that r = f ( θ ) {r}={f{{\left(\theta\right)}}} r = f ( θ ) r {r} r θ \theta θ
To find slope of tangent line, we need to find d y d x \frac{{{d}{y}}}{{{d}{x}}} d x d y r {r} r θ \theta θ
d y d x = d y d θ ⋅ d θ d x = d y d θ ⋅ 1 d x d θ \frac{{{d}{y}}}{{{d}{x}}}=\frac{{{d}{y}}}{{{d}\theta}}\cdot\frac{{{d}\theta}}{{{d}{x}}}=\frac{{{d}{y}}}{{{d}\theta}}\cdot\frac{{1}}{{\frac{{{d}{x}}}{{{d}\theta}}}} d x d y = d θ d y ⋅ d x d θ = d θ d y ⋅ d θ d x 1
We have that d y d θ = d r d θ sin ( θ ) + r cos ( θ ) \frac{{{d}{y}}}{{{d}\theta}}=\frac{{{d}{r}}}{{{d}\theta}}{\sin{{\left(\theta\right)}}}+{r}{\cos{{\left(\theta\right)}}} d θ d y = d θ d r sin ( θ ) + r cos ( θ ) d x d θ = d r d θ cos ( θ ) − r sin ( θ ) \frac{{{d}{x}}}{{{d}\theta}}=\frac{{{d}{r}}}{{{d}\theta}}{\cos{{\left(\theta\right)}}}-{r}{\sin{{\left(\theta\right)}}} d θ d x = d θ d r cos ( θ ) − r sin ( θ )
So, we obtained following fact.
Fact. Slope of tangent line in polar coordinates is d y d x = d r d θ sin ( θ ) + r cos ( θ ) d r d θ cos ( θ ) − r sin ( θ ) \frac{{{d}{y}}}{{{d}{x}}}=\frac{{\frac{{{d}{r}}}{{{d}\theta}}{\sin{{\left(\theta\right)}}}+{r}{\cos{{\left(\theta\right)}}}}}{{\frac{{{d}{r}}}{{{d}\theta}}{\cos{{\left(\theta\right)}}}-{r}{\sin{{\left(\theta\right)}}}}} d x d y = d θ d r c o s ( θ ) − r s i n ( θ ) d θ d r s i n ( θ ) + r c o s ( θ )
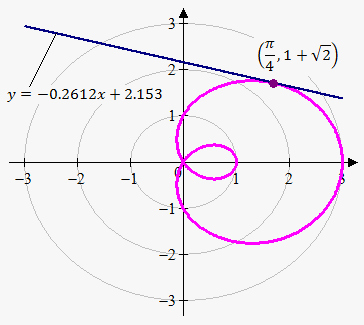
Example. Find equation of tangent line to r = 1 + 2 cos ( θ ) {r}={1}+{2}{\cos{{\left(\theta\right)}}} r = 1 + 2 cos ( θ ) θ = π 4 \theta=\frac{\pi}{{4}} θ = 4 π
d r d θ = − 2 sin ( θ ) \frac{{{d}{r}}}{{{d}\theta}}=-{2}{\sin{{\left(\theta\right)}}} d θ d r = − 2 sin ( θ )
d y d x = − 2 sin ( θ ) sin ( θ ) + ( 1 + 2 cos ( θ ) ) cos ( θ ) − 2 sin ( θ ) cos ( θ ) − ( 1 + 2 cos ( θ ) ) sin ( θ ) = \frac{{{d}{y}}}{{{d}{x}}}=\frac{{-{2}{\sin{{\left(\theta\right)}}}{\sin{{\left(\theta\right)}}}+{\left({1}+{2}{\cos{{\left(\theta\right)}}}\right)}{\cos{{\left(\theta\right)}}}}}{{-{2}{\sin{{\left(\theta\right)}}}{\cos{{\left(\theta\right)}}}-{\left({1}+{2}{\cos{{\left(\theta\right)}}}\right)}{\sin{{\left(\theta\right)}}}}}= d x d y = − 2 s i n ( θ ) c o s ( θ ) − ( 1 + 2 c o s ( θ ) ) s i n ( θ ) − 2 s i n ( θ ) s i n ( θ ) + ( 1 + 2 c o s ( θ ) ) c o s ( θ ) =
= cos ( θ ) + 2 cos ( 2 θ ) − sin ( θ ) − 2 sin ( 2 θ ) =\frac{{{\cos{{\left(\theta\right)}}}+{2}{\cos{{\left({2}\theta\right)}}}}}{{-{\sin{{\left(\theta\right)}}}-{2}{\sin{{\left({2}\theta\right)}}}}} = − s i n ( θ ) − 2 s i n ( 2 θ ) c o s ( θ ) + 2 c o s ( 2 θ )
So, slope of tangent line at point θ = π 4 \theta=\frac{\pi}{{4}} θ = 4 π m = d y d x ∣ θ = π 4 = cos ( π 4 ) + 2 cos ( π 2 ) − sin ( π 4 ) − 2 sin ( π 2 ) = 1 7 ( 1 − 2 2 ) {m}=\frac{{{d}{y}}}{{{d}{x}}}{\mid}_{{\theta=\frac{\pi}{{4}}}}=\frac{{{\cos{{\left(\frac{\pi}{{4}}\right)}}}+{2}{\cos{{\left(\frac{\pi}{{2}}\right)}}}}}{{-{\sin{{\left(\frac{\pi}{{4}}\right)}}}-{2}{\sin{{\left(\frac{\pi}{{2}}\right)}}}}}=\frac{{1}}{{7}}{\left({1}-{2}\sqrt{{{2}}}\right)} m = d x d y ∣ θ = 4 π = − s i n ( 4 π ) − 2 s i n ( 2 π ) c o s ( 4 π ) + 2 c o s ( 2 π ) = 7 1 ( 1 − 2 2 )
Now we need corresponding x {x} x y {y} y θ = π 4 \theta=\frac{\pi}{{4}} θ = 4 π
x = r cos ( θ ) = ( 1 + 2 cos ( θ ) ) cos ( θ ) = ( 1 + 2 cos ( π 4 ) ) cos ( π 4 ) = 1 + 2 2 {x}={r}{\cos{{\left(\theta\right)}}}={\left({1}+{2}{\cos{{\left(\theta\right)}}}\right)}{\cos{{\left(\theta\right)}}}={\left({1}+{2}{\cos{{\left(\frac{\pi}{{4}}\right)}}}\right)}{\cos{{\left(\frac{\pi}{{4}}\right)}}}=\frac{{{1}+\sqrt{{{2}}}}}{\sqrt{{{2}}}} x = r cos ( θ ) = ( 1 + 2 cos ( θ ) ) cos ( θ ) = ( 1 + 2 cos ( 4 π ) ) cos ( 4 π ) = 2 1 + 2
y = r sin ( θ ) = ( 1 + 2 cos ( θ ) ) sin ( θ ) = ( 1 + 2 cos ( π 4 ) ) sin ( π 4 ) = 1 + 2 2 {y}={r}{\sin{{\left(\theta\right)}}}={\left({1}+{2}{\cos{{\left(\theta\right)}}}\right)}{\sin{{\left(\theta\right)}}}={\left({1}+{2}{\cos{{\left(\frac{\pi}{{4}}\right)}}}\right)}{\sin{{\left(\frac{\pi}{{4}}\right)}}}=\frac{{{1}+\sqrt{{{2}}}}}{\sqrt{{{2}}}} y = r sin ( θ ) = ( 1 + 2 cos ( θ ) ) sin ( θ ) = ( 1 + 2 cos ( 4 π ) ) sin ( 4 π ) = 2 1 + 2
So, equation of tangent line that passes through point θ = π 4 \theta=\frac{\pi}{{4}} θ = 4 π y = 1 7 ( 1 − 2 2 ) ( x − 1 + 2 2 ) + 1 + 2 2 {y}=\frac{{1}}{{7}}{\left({1}-{2}\sqrt{{{2}}}\right)}{\left({x}-\frac{{{1}+\sqrt{{{2}}}}}{\sqrt{{{2}}}}\right)}+\frac{{{1}+\sqrt{{{2}}}}}{\sqrt{{{2}}}} y = 7 1 ( 1 − 2 2 ) ( x − 2 1 + 2 ) + 2 1 + 2 y = − 0.2612 x + 2.153 {y}=-{0.2612}{x}+{2.153} y = − 0.2612 x + 2.153
As with parametric curves there are curves that have several tangent line at one point. For example, in above example, such point is (0,0). The corresponding value(s) of θ \theta θ 1 + 2 cos ( θ ) = 0 {1}+{2}{\cos{{\left(\theta\right)}}}={0} 1 + 2 cos ( θ ) = 0 [ 0 , 2 π ] {\left[{0},{2}\pi\right]} [ 0 , 2 π ] 2 π 3 \frac{{{2}\pi}}{{3}} 3 2 π 4 π 3 \frac{{{4}\pi}}{{3}} 3 4 π
Similarly to parametric curves, curve in polar coordinates has
horizontal tangent when d y d θ = 0 \frac{{{d}{y}}}{{{d}\theta}}={0} d θ d y = 0 d x d θ ≠ 0 \frac{{{d}{x}}}{{{d}\theta}}\ne{0} d θ d x = 0 vertical tangent when d x d θ = 0 \frac{{{d}{x}}}{{{d}\theta}}={0} d θ d x = 0 d y d θ ≠ 0 \frac{{{d}{y}}}{{{d}\theta}}\ne{0} d θ d y = 0
We have that , so