Hydrostatic Pressure and Force
Suppose that a thin horizontal plate with area $$${A}$$$ square meters is submerged in a fluid of density $$$\rho$$$ kilograms per cubic meter at a depth $$${d}$$$ meters below the surface of the fluid.
The volume of fluid above plate is $$${V}={A}{d}$$$, so its mass is $$${m}=\rho{V}=\rho{A}{d}$$$.
The force exerted by the fluid on the plate is $$${F}={m}{g{=}}\rho{g{{A}}}{d}$$$ where $$${g{=}}{9.8}\frac{{m}}{{{s}}^{{2}}}$$$.
The pressure on the plate is defined to be the force per unit area: $$${P}=\frac{{F}}{{A}}=\rho{g{{d}}}$$$.
The SI unit for measuring pressure is newtons per square meter, which is called a pascal $$$\left({1}\frac{{N}}{{{m}}^{{2}}}={1}{P}{a}\right)$$$. Since this is a small unit, the kilopascal (kPa) is often used.
For example, since density of water is $$$\rho={1000}\frac{{{k}{g}}}{{{m}}^{{3}}}$$$, the pressure at the bottom of a swimming pool 3 meters deep is $$${P}=\rho{g{{d}}}={1000}\frac{{{k}{g}}}{{{m}}^{{3}}}\times{9.8}\frac{{m}}{{{s}}^{{2}}}\times{3}{m}={29400}{P}{a}={29.4}\ {k}{P}{a}$$$.
So we can determine the hydrostatic force against a vertical plate or wall or dam in a fluid. We again must use integrals, because the pressure is not constant but increases as the depth increases.
Example 1. Suppose we have a trapezoidal dam whose height is 15m. Lower base is 20 m and upper base is 30 m. Find the force on the dam due to hydrostatic pressure if the water level is 5 m from the top of the dam.
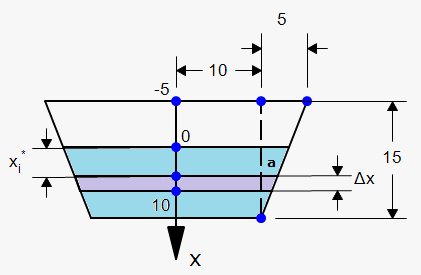
We need convention for $$${x}$$$. Let $$${x}={0}$$$ corresponds to the surface of water and $$${x}={10}$$$ be bottom of the dam. Then water is in interval $$${\left[{0},{10}\right]}$$$.
Now divide interval $$${\left[{0},{10}\right]}$$$ into $$${n}$$$ subintervals of equal length with endpoints $$${x}_{{i}}$$$ and we choose $$${{x}_{{i}}^{{\star}}}\in{\left[{x}_{{{i}-{1}}},{x}_{{i}}\right]}$$$. The i-th horizontal strip of the dam is approximated by a rectangle with height $$$\Delta{x}$$$ and width $$${w}_{{i}}$$$,
To find $$${w}_{{i}}$$$ we need to find $$${a}$$$ first.
From similar triangle we have that $$$\frac{{a}}{{{10}-{{x}_{{i}}^{{\star}}}}}=\frac{{5}}{{15}}$$$ or $$${a}=\frac{{10}}{{3}}-\frac{{1}}{{3}}{{x}_{{i}}^{{\star}}}$$$.
Therefore, $$${w}_{{i}}={2}{\left({10}+{a}\right)}={2}{\left({10}+\frac{{10}}{{3}}-\frac{{1}}{{3}}{{x}_{{i}}^{{\star}}}\right)}=\frac{{80}}{{3}}-\frac{{2}}{{3}}{{x}_{{i}}^{{\star}}}$$$.
If $$${A}_{{i}}$$$ is the area of the i-th strip then $$${A}_{{i}}\approx{w}_{{i}}\Delta{x}={\left(\frac{{80}}{{3}}-\frac{{2}}{{3}}{{x}_{{i}}^{{\star}}}\right)}\Delta{x}$$$.
If $$$\Delta{x}$$$ is small then pressure $$${P}_{{i}}$$$ is almost constant, so $$${P}_{{i}}\approx\rho{{g{{x}}}_{{i}}^{{\star}}}={1000}\cdot{9.8}{{x}_{{i}}^{{\star}}}={9800}{{x}_{{i}}^{{\star}}}$$$.
The hydrostatic force acting on the i-th strip is the product of the pressure and the area: $$${F}_{{i}}\approx{P}_{{i}}{A}_{{i}}\approx{9800}{{x}_{{i}}^{{\star}}}{\left(\frac{{80}}{{3}}-\frac{{2}}{{3}}{{x}_{{i}}^{{\star}}}\right)}\Delta{x}$$$.
Adding these forces and taking the limit as $$${n}\to\infty$$$, we obtain the total hydrostatic force on the dam: $$${F}=\lim_{{{n}\to\infty}}{\sum_{{{i}={1}}}^{{n}}}{9800}{{x}_{{i}}^{{\star}}}{\left(\frac{{80}}{{3}}-\frac{{2}}{{3}}{{x}_{{i}}^{{\star}}}\right)}\Delta{x}={9800}{\int_{{0}}^{{{10}}}}{x}{\left(\frac{{80}}{{3}}-\frac{{2}}{{3}}{x}\right)}{d}{x}={9800}{\int_{{0}}^{{{10}}}}{\left(\frac{{80}}{{3}}{x}-\frac{{2}}{{3}}{{x}}^{{2}}\right)}{d}{x}=$$$
$$$={9800}{\left(\frac{{40}}{{3}}{{x}}^{{2}}-\frac{{2}}{{9}}{{x}}^{{3}}\right)}{{\mid}_{{0}}^{{{10}}}}\approx{1.09}\times{{10}}^{{7}}\ {N}$$$.
Example 2. Find force due to hydrostatic pressure on circular plate of radius 4 that is 3 meters under water.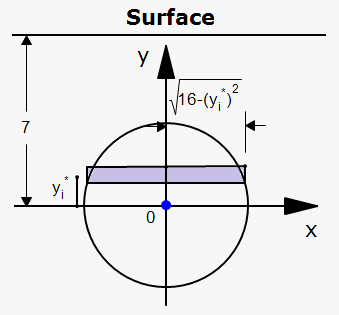
Again we need convention about $$${x}$$$. This time we will take origin to be at the center of circle and $$${x}$$$ pointing to the right (standard rectangular system).
Now we split plate by $$${n}$$$ horizontal strips of heigth $$$\Delta{y}$$$ and choose sample point $$${{y}_{{i}}^{\star}}$$$ from i-th strip.
We can approximate area of i-th strip by rectangle with height $$$\Delta{y}$$$ and width $$${2}\sqrt{{{16}-{{\left({{y}_{{i}}^{\star}}\right)}}^{{2}}}}$$$: $$${A}_{{i}}\approx{2}\sqrt{{{16}-{{\left({{y}_{{i}}^{\star}}\right)}}^{{2}}}}{d}{y}$$$.
i-strip is $$${7}-{{y}_{{i}}^{\star}}$$$ meters under water the pressure on each strip is $$${P}_{{i}}\approx\rho\cdot{g{\cdot}}{\left({7}-{{y}_{{i}}^{\star}}\right)}={9800}{\left({7}-{{y}_{{i}}^{\star}}\right)}$$$.
Now force that acts on each strip is $$${F}_{{i}}\approx{P}_{{i}}{A}_{{i}}={19600}{\left({7}-{{y}_{{i}}^{\star}}\right)}\sqrt{{{16}-{{\left({{y}_{{i}}^{\star}}\right)}}^{{2}}}}{d}{y}$$$.
If we now add force that acts on each strip and take limit $$${n}\to\infty$$$ then we will obtain that $$${F}={19600}{\int_{{-{4}}}^{{4}}}{\left({7}-{y}\right)}\sqrt{{{16}-{{y}}^{{2}}}}{d}{y}={19600}{\left({\int_{{-{4}}}^{{4}}}{7}\sqrt{{{16}-{{y}}^{{2}}}}{d}{y}-{\int_{{-{4}}}^{{4}}}{y}\sqrt{{{16}-{{y}}^{{2}}}}\right)}{d}{y}$$$.
First interval requires trigonometric substitution $$${y}={4}{\sin{{\left({u}\right)}}}$$$, second requires substitution $$${v}={16}-{{y}}^{{2}}$$$.
So, $$${F}={1097600}\pi$$$.
